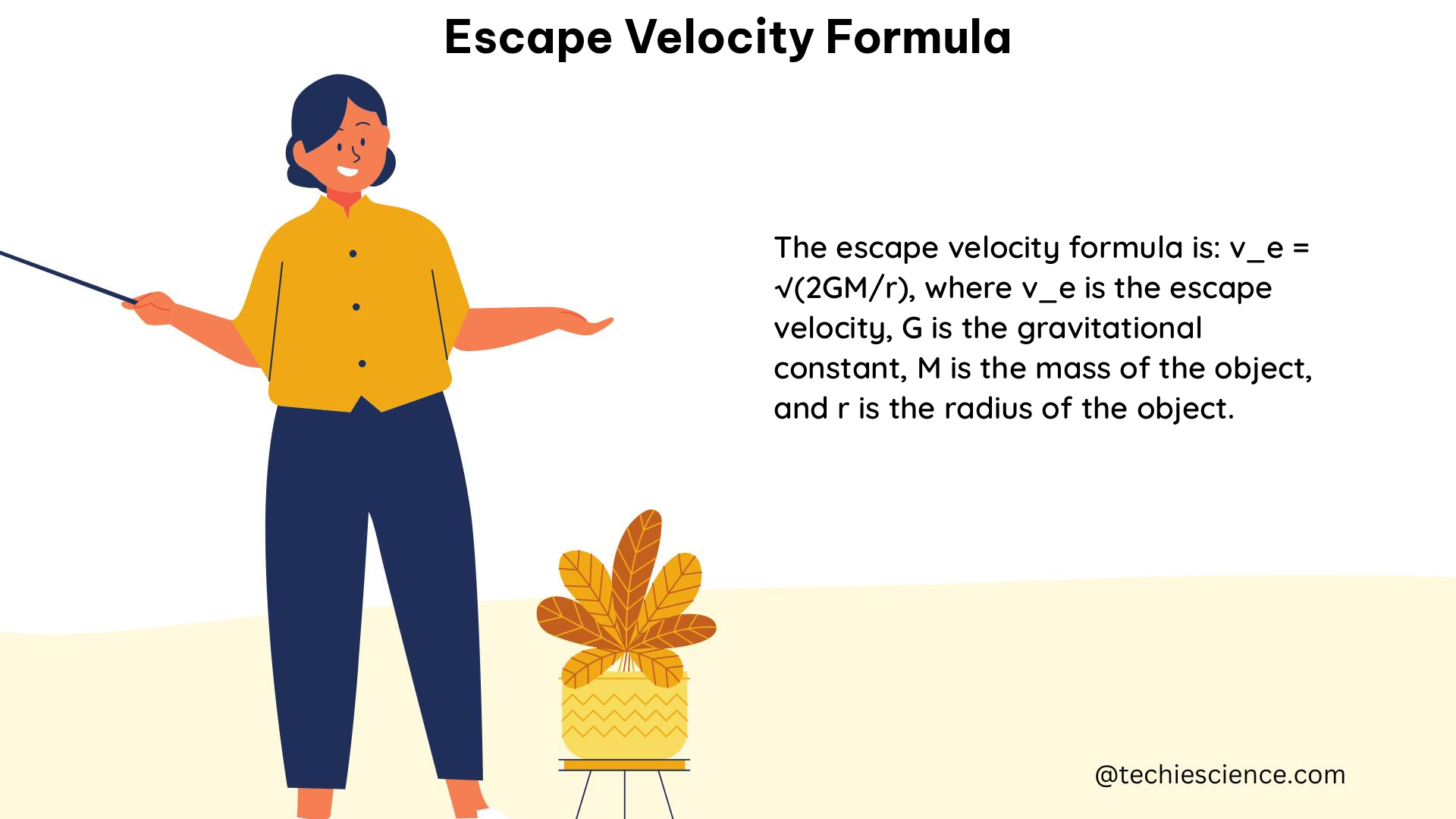
The Escape Velocity Formula: A Comprehensive Guide for Physics Students

by

The escape velocity formula, v_esc = √(2GM/r), is a fundamental concept in astrophysics and space exploration, used to calculate the minimum speed required for an object to break free from the gravitational field of a celestial body without further propulsion. This formula is derived from the law of conservation of energy and is independent of the properties of the escaping object, relying solely on the mass and radius of the celestial body.

Understanding the Escape Velocity Formula

The escape velocity formula is composed of four key variables:

  1. v_esc: The escape velocity, or the minimum speed an object must attain to escape the gravitational pull of a celestial body.
  2. G: The gravitational constant, which has a value of approximately 6.674 × 10^-11 N⋅m^2/kg^2.
  3. M: The mass of the celestial body.
  4. r: The radius of the celestial body.

The formula can be derived from the law of conservation of energy, which states that the total energy of an object must remain constant. At the moment of launch, an object has both potential energy (PE) and kinetic energy (KE). When the object finally escapes, its potential energy is equal to zero, and its kinetic energy is also equal to zero. Therefore, the initial total energy (TE) of the object must be equal to zero.

The mathematical derivation of the formula is as follows:

0 = -PE + KE
0 = -(GMm/r) + (1/2)mv^2

Solving for v, we get:

v = √(2GM/r)

This formula is independent of the properties of the escaping object, such as its mass or composition, and only depends on the mass and radius of the celestial body.

Escape Velocity Calculations for Planets and Moons

escape velocity formula

Using the escape velocity formula, we can calculate the typical escape velocities for the planets and moons in our solar system. These values are as follows:

Celestial Body Escape Velocity (km/s)
Mercury 4.3
Venus 10.3
Earth 11.2
Moon 2.4
Mars 5.0
Jupiter 59.6
Saturn 35.6
Uranus 21.3
Neptune 23.8

These values can be calculated using the escape velocity formula and the known masses and radii of the respective celestial bodies.

Practical Applications of the Escape Velocity Formula

The escape velocity formula has numerous practical applications in the field of space exploration and astrophysics, including:

  1. Spacecraft Design: The escape velocity formula is crucial in determining the minimum speed required for a spacecraft to break free from the gravitational pull of a planet or moon, allowing for the design of efficient and effective propulsion systems.

  2. Satellite Orbits: The formula is used to calculate the minimum velocity required for a satellite to achieve a stable orbit around a celestial body, ensuring that the satellite does not fall back to the surface or escape the gravitational field.

  3. Asteroid and Comet Deflection: The escape velocity formula can be used to determine the amount of force or energy required to deflect or redirect an asteroid or comet that poses a threat to Earth, potentially preventing a catastrophic collision.

  4. Stellar and Galactic Escape: The formula can be applied to the study of stellar and galactic escape, helping astrophysicists understand the dynamics of matter and energy within the universe.

  5. Gravitational Slingshot Maneuvers: The escape velocity formula is crucial in the design of gravitational slingshot maneuvers, where a spacecraft uses the gravity of a planet or moon to gain a boost in velocity and change its trajectory.

Numerical Examples and Problem-Solving

To further illustrate the application of the escape velocity formula, let’s consider a few numerical examples:

  1. Example 1: Calculate the escape velocity of a spacecraft from the surface of the Earth.
    Given:
  2. Mass of the Earth (M) = 5.972 × 10^24 kg

  3. Radius of the Earth (r) = 6,371 km
    Using the formula:
    v_esc = √(2GM/r)
    v_esc = √(2 × 6.674 × 10^-11 × 5.972 × 10^24 / 6.371 × 10^3)
    v_esc = 11.2 km/s

  4. Example 2: Determine the minimum speed required for a spacecraft to escape the gravitational field of the Moon.
    Given:

  5. Mass of the Moon (M) = 7.342 × 10^22 kg

  6. Radius of the Moon (r) = 1,737 km
    Using the formula:
    v_esc = √(2GM/r)
    v_esc = √(2 × 6.674 × 10^-11 × 7.342 × 10^22 / 1.737 × 10^3)
    v_esc = 2.4 km/s

  7. Example 3: Calculate the escape velocity from the surface of Jupiter.
    Given:

  8. Mass of Jupiter (M) = 1.899 × 10^27 kg
  9. Radius of Jupiter (r) = 69,911 km
    Using the formula:
    v_esc = √(2GM/r)
    v_esc = √(2 × 6.674 × 10^-11 × 1.899 × 10^27 / 69.911 × 10^3)
    v_esc = 59.6 km/s

These examples demonstrate the versatility of the escape velocity formula and its application in various space exploration scenarios.

Conclusion

The escape velocity formula, v_esc = √(2GM/r), is a fundamental concept in astrophysics and space exploration, used to calculate the minimum speed required for an object to break free from the gravitational field of a celestial body without further propulsion. This formula is derived from the law of conservation of energy and is independent of the properties of the escaping object, relying solely on the mass and radius of the celestial body.

By understanding the escape velocity formula and its practical applications, physics students can gain valuable insights into the dynamics of space exploration, satellite orbits, asteroid and comet deflection, and the broader study of stellar and galactic escape. Through the use of numerical examples and problem-solving, students can further develop their skills in applying this formula to real-world scenarios, preparing them for the challenges and advancements in the field of astrophysics and space exploration.

References:

  1. Escape Velocity Formula: Derivation and Examples
  2. Escape Velocity: Definition, Formula, and Calculations
  3. Escape Velocity: Concept and Calculation
  4. Escape Velocity and the Conservation of Energy