3 Important Theorems On Electrical Circuit Analysis

Points of Discussion: Electrical Circuit Analysis

• Introduction to advanced Electrical Circuit Analysis
• Thevenin’s Theorem
• Norton’s Theorem
• Superposition Theorem

Introduction to Advanced Electrical Circuit Analysis

We came to know the primary circuit structure and some essential terminologies in the previous circuit analysis article. In the DC Circuit analysis, we have studied KCL, KVL. In this article, we are going to learn about some advanced methods for circuit analysis. They are – Superposition theorem, Thevenin’s theorem, Norton’s theorem. There are many more methods for circuit analysis like – maximum power transfer theory, Millman’s theory, etc.

We will learn about the theory of the methods, the detailed explanation of the theory and steps for solving circuit problems.

Basic Terminologies Related to Circuit Analysis : Click Here!

Advanced Electrical Circuit Analysis: Thevenin’s Theorem

Thevenin’s theorem (Helmholtz – Thevenin theorem) is one of the most crucial theories needed for analysing and studying complex circuits. It is one of the simplest methods to solve complex network problems. Also, it is one of the most widely used methods for circuit analysis.

Thevenin’s Theorem: It states that all complex networks can be replaced by a voltage source and a resistance in series connection.

In simpler words, if a circuit has energy sources like dependent or independent voltage sources, and has a complex structure of resistances, then the whole circuit is representable as a circuit consisting the equivalent voltage source, the load resistance, and the equivalent resistance of the circuit, all in series connection.

Steps for solving problems regarding Thevenin’s theorem

• Step 1: Remove the Load Resistance and redraw the circuit. (Note: The load resistance will be the referenced resistance through which you have to calculate the current).
• Step 2: Find out the open circuit voltage or Thevenin’s equivalent voltage for the circuit.
• Step 3: Now short circuit all the voltage sources, and open circuit all the current sources. Also, substitute all the elements with their equivalent resistances and redraw the circuit (Note: Keep the load resistance is unattached).
• Step 4: Find out the equivalent resistance of the circuit.
• Step 5: Draw a fresh circuit with a voltage source and two resistance in series with it. The magnitude of the voltage source will be the same as the derived equivalent Thevenin’s voltage. One of the resistances will be the pre-calculated equivalent resistance, and the other is the load resistance.
• Step 6: Calculate the current through the circuit. That is the final answer.

Explanation

To explain the theorem, let us take a complex circuit as below.

In this circuit, we have to find out the current I, through the resistance RL using the Thevenin’s theorem.

Now, to do so, first remove the load resistance and make that branch open circuited. Find out the open circuit voltage or Thevenin’s equivalent across that branch. The open circuited voltage comes as: V_OC = I R₃ = (V_S / R₁ + R₃) R₃

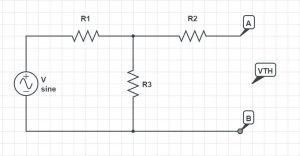
For calculation of the equivalent resistance, the voltage source is short circuited (deactivated). Now, find out the resistance. The equivalent resistance comes out as: R_TH = R₂ + [(R₁ R₃) / (R₁ + R₃)]

At the last step, make a circuit using the derived equivalent voltage and equivalent resistance. Connect the load resistance in series with the equivalent resistance. 

The current comes as: I_L = V_TH / (R_TH + R_L)

Electrical Circuit Analysis: Norton’s Theorem

Norton’s theorem (Mayer – Norton Theorem) is another crucial theory needed to analyses and study complex circuits. It is one of the simplest methods to solve complex network problems. Also, it is one of the most widely used methods for circuit analysis.

Norton’s Theorem: It states that all complex networks can be replaced by a current source and a resistance in parallel connection.

In simpler words, if a circuit has energy sources like dependent or independent current sources, and has a complex structure of resistances, then the whole circuit is representable as a circuit consisting the equivalent current source, the load resistance, and the equivalent resistance of the circuit, all in parallel connection.

Steps for solving problems regarding Norton’s theorem

• Step 1: Short circuit the Load Resistance and redraw the circuit. (Note: The load resistance will be the referenced resistance through which you have to calculate the current).
• Step 2: Find out the short circuit current or Norton’s current of the circuit.
• Step 3: Now, short circuit all the independent sources. Also, substitute all the elements with their equivalent resistances and redraw the circuit (Note: Make the load resistance unattached).
• Step 4: Find out the equivalent resistance of the circuit.
• Step 5: Draw a fresh circuit with a current source and two resistance in parallel with it. The magnitude of the current source will be the same as the derived equivalent short-circuit current. One of the resistances will be the pre-calculated equivalent resistance, and the other is the load resistance.
• Step 6: Calculate the current through the circuit. That is the final answer.

Explanation

To explain the theorem, let us take a complex circuit as below.

In this circuit, we have to find out the current I, through the resistance RL using Norton’s theorem.

To do so, first, remove the load resistance (R_L) and make that branch short circuited. The current in the closed loop is calculated first.

I = V_S / [ R₁ + {R₂R₃/ (R₂ + R₃)}]

The short circuit current comes as I_SC = I R₃ / (R₃ + R₂)

The voltage source is short circuited (deactivated) and the load resistance branch is short circuited for calculation of the equivalent resistance. Now, find out the resistance. The equivalent resistance comes out as: R_NT = R₂ + [(R₁ R₃) / (R₁ + R₃)]

At the last step, make a circuit using the derived equivalent current source and equivalent resistance. Connect the load resistance in parallel with the equivalent resistance and the current source in parallel with them. 

The current comes as: I_L = I_SC R_NT / (R_NT + R_L)

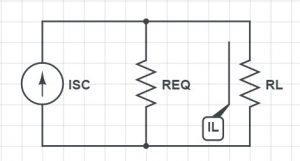
Electrical Circuit Analysis: Superposition Theorem

Superposition theorem is another crucial theory needed for analysing and studying of complex circuits. It is another easy method to solve complex network problems. Also, it is one of the most widely used methods for circuit analysis. Superposition theory is only applicable for linear circuits and circuits which obey Ohm’s law.

Superposition Theorem: It states that for all active, linear circuits, which have multiple sources, the response across any circuit element, is the aggregate sum of the responses obtained from each source considered separately and every source are substituted by their internal resistances.

In a more general way, the theorem states that the aggregate current in each branch can be expressed as the sum of all currents produced for a linear network. At the same time, all the source acted separately, and their internal resistances substitute independent sources.

Steps for solving problems regarding superposition theorem

• Step 1: Consider one independent source at a time and deactivate (short-circuit) all the other sources.
• Step 2:  Replace that other source with the equivalence of the resistors of the circuits. (Note: By default, if the resistance is not given, make it short-circuit).
• Step 3: Now, short circuit all the other (leave the selected source) voltage source and open circuit all the other current source. 
• Step 4: Find the current for every branch of the circuit.
• Step 5: Now choose another voltage source and follow step 1-4. Please do it for every independent source.
• Step 6: At last, calculate the current for each branch by superposition theorem (addition). To do so, add up currents of the same branch calculated for different voltage sources. Add the direction of the currents wise (if the same direction – add up, else minus).

Explanation

To explain the method, let us take a complex circuit as below.

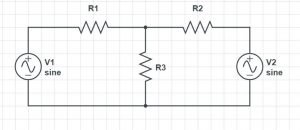
In this circuit, we have to find out the current through each branch. The circuit has two voltage sources.

At first, we choose the V₁ source. So, we short circuit (as the source’s internal resistance is not given) the other voltage source – V₂.

Now, calculate all the current for every branch. Let the current through the branches are – I₁`, I<sub>2</sub>`, I₃`. They are represented as follow.

I₁` = V₁ / [ R₁ + {R₂R₃/ (R₂ + R₃)}]

I₂` = I<sub>1</sub>` R₃ / (R₃ + R₂)

Now, I₃` = I<sub>1</sub>` – I₂`

The V₂ voltage source is activated in the next step while the V₁ source is deactivated or short circuited (internal resistance is not given).

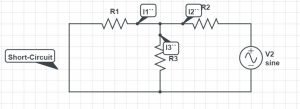
As the previous step, here we need to calculate the current for every branch again. The current through the branches comes as follow.

I₂“ = V₂ / [ R₂ + {R₁R₃/ (R₁ + R₃)}]

I₁“ = I₂“ R₃ / (R₃ + R₁)

Now, I₃“ = I₂“ – I₁“

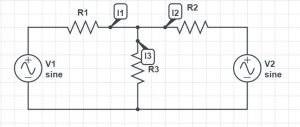
All the source calculation is now covered. Now, we have to apply superposition theorem and find out the net currents for the branches. The direction rule is considered while calculating. The I₁, I₂, I₃ magnitudes are given below.

I₃ = I₃` + I₃“

I₂ = I₂` – I₂“

I₁ = I₁` – I₁“

For mathematical problems, check out the next article.