Bulk Modulus: 25 Facts You Should Know

by

Bulk Modulus Definition:

Bulk modulus is the ability of the material to be resistant to compression.
It is the volumetric elasticity and is inversely proportional to Compressibility. The object having incompressibility deform in all directions when the load is applied from all directions.

Bulk modulus is the volumetric stress over volumetric strain.

The ratio of the increase in pressure relative to the decrease in volume.

Bulk modulus representation:

bulk modulus
Image credit:AG Caesar, Isostatic pressure deformation, CC0 1.0

Bulk modulus symbol:
K or B

Bulk modulus equation:


K=-V\\frac{dP}{dV}
where

P=pressure
V=initial volume
dP/dV = derivative of pressure with respect to volume.
V=\\frac{m}{\\rho}
Hence,

K=\\rho \\frac{dP}{d\\rho}

Bulk modulus unit:


SI unit of Bulk modulus of elasticity: N/m^2(Pa)

Dimension of Bulk modulus:

[M^{1}L^{-1}T^{-2}]

Bulk modulus pressure:


The effect of pressure on incompressibility explained from the below graph:

2

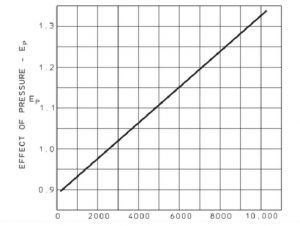
The value of Bulk modulus is required to determine:


The value of the Bulk modulus is required to determine the Mach number.
Mach number is a dimensionless quantity.

Bulk modulus measurement:


How incompressible a solid is measured by the Bulk modulus. Hence bulk modulus also referred as incompressibility.

The incompressibility of fluid:


The fluid volume modulus is the measure of resistance to compression.
It is the ratio of fluid volume stress to volumetric strain.

Bulk modulus of various materials:

Materials : Bulk modulus values

The Bulk modulus of water:2.2Gpa

The Bulk modulus of water at high pressure:2.1Gpa

The Bulk modulus of air:142Kpa isentropic, 101Kpa isothermal

Bulk modulus for steel:160Gpa

Bulk modulus of mineral oil:1.8Gpa

Bulk modulus of mercury:28.5Gpa

The adiabatic Bulk modulus of air:142Kpa

The Bulk modulus of diesel:1.477Gpa (at 6.89Mpa and 37.8°C)

The Bulk modulus of ice:11-8.4Gpa(0K-273K)

The Bulk modulus of hydraulic oil:

The Bulk modulus of concrete:30-50Gpa

The Bulk modulus of diamond:443Gpa

The Bulk modulus of rubber:1.5-2Gpa

The Bulk modulus of water at high pressure:2-5Gpa

330px SpiderGraph BulkModulus
Image credit:Afluegel at English Wikipedia, SpiderGraph BulkModulus, marked as public domain, more details on Wikimedia Commons

Hydraulic fluid incompressibility:


Hydraulic fluid incompressibility is the Compressibility resistant property of the material.
Hydraulic fluid gets affected by the applied pressure.
As the applied pressure increases, the volume of the body decreases.


The Bulk modulus of elasticity:


The modulus of elasticity of liquid varies depending on the specific gravity and the temperature of the liquid.
K is always constant within elastic limit of the material.
This is the Bulk modulus of elasticity.

K=Volumetric stress/volumetric strain
K=-V\\frac{dP}{dV}

The sign indicates the decrease in volume.

Volume modulus is associated with a change in volume.

Compressibility is calculated as reciprocal of incompressibility.
Compressibility represented as,
Compressibility=1/K
SI unit: m^2/N or Pa^-1.
Dimensions of Compressibility: [M^-1L^-1T^2]

Derivation of Bulk modulus of elasticity:

Bulk fluid modulus is the ratio of the change in pressure to change in volumetric strain.
\\frac {-\\delta V} {V}=\\frac {\\delta P} {K}
δV: change in volume
δp: change in pressure
V: actual volume
K: volume modulus
δp tends to zero
K=-V\\frac{dp}{dv}
V=1/density
Vd\\rho +\\rho dV=0
dV=-(\\frac{V}{\\rho}) d\\rho
dV=\\frac{-Vdp}{-(\\frac{V}{\\rho}) d\\rho}

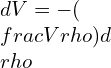
The Bulk modulus of incompressible liquid:

The volume of incompressible fluid does not change. As the force is applied, the change in volume is zero due to the volumetric strain of the incompressible fluid is zero.

Temperature dependence:

The modulus of incompressibility evolves due to the volumetric stress evolves periodically.

It is coupled to shear modulus, Assume constant Poisson’s ratio.

K=\\frac{2\\mu (1+\ u )}{3(1-2\ u )}

The time-dependent modulus is represented as,

k(t)=[\\frac{2\\mu (t)[1+\ u}{3(1-2\ u )}]

Elastic constants relationships:


Relationships between Poisson’s ratio, Young’s modulus and shear modulus with bulk modulus:

Young’s modulus, Poisson’s ratio:
Elastic modulus, Shear modulus:
E=3K(1-2μ)
G=3KE/9K-E
K= EG/3(3G-E)
K= E/3(1-2μ)
K=2G(1+μ)/3(1-2μ)

For an incompressible fluid, the maximum limit of poisson’s ratio be 0.5.
For K to be positive μ should be always than 0.5.
n = 0.5.
3G = E.
K = ∞.
E= 3K(1-2 μ)
E= 2G(1+μ)
2G(1+μ)=3K(1-2 μ)

Distinguish between young’s modulus and bulk modulus:


Young’s modulus is related with longitudinal stress and longitudinal strain of the body.

Incompressibility is the form of volumetric stress and volumetric strain.
Bulk modulus exists in solid, liquid and gas, whereas Young’s modulus exists in only solids.
Young’s modulus gives the change in length of the body, whereas Bulk modulus gives the change in volume of the body.

Distinguish between Shear modulus and Bulk modulus :


Bulk modulus is the form of volumetric stress and volumetric strain. It involves the effect of the applied pressure. as the pressure increase , the volume of the body decreases. This gives the negative sign to the ratio of the stress to strain. The ratio is associated with the volume of the body.
In case of shear modulus, shear modulus is the form of shearing stress and shearing strain. It involves the effect of shear stress on the body. It is the response to the deformation of the body. The ratio is associated with the shape of the body.
G=\\frac{\\tau }{A}
G=\\frac{\\frac{F}{A}}{tan\\Theta }

where,
T=shear stress
gamma=shear strain
Incompressibility exists in solid, liquid and gas, whereas shear modulus exists in only solids.

Isentropic Bulk modulus:


Incompressibility of the body at the constant entropy is called isentropic bulk modulus.
The ratio of change in applied pressure to fractional volume change in the body due to the pressure change is a form of the isentropic incompressibility.

Isothermal Bulk modulus:


When the temperature is constant throughout the incompressibility is called isothermal bulk modulus.
The ratio of change in applied pressure to fractional volume change in the body due to the pressure change is a form of the isentropic incompressibility

Negative Bulk modulus:

Why negative:

Bulk modulus has a negative sign because of the decrease in volume due to an increase in pressure.

Adiabatic Bulk modulus:


Adiabatic Bulk modulus is the ratio of the pressure to change in fractional volume in the adiabatic process when there is no heat exchange with the surrounding.

PV^{\\gamma }=const

It is represented as,

K=-\\frac{dP}{\\frac{dV}{V}}
Where γ= ratio of specific heats.

The ratio of adiabatic to isothermal Bulk modulus:
\\gamma =\\frac{C_{p}}{C_{v}}

Adiabatic incompressibility is the modulus in the adiabatic process.
Isothermal incompressibility is the modulus is at a constant temperature.
Hence the ratio of the Adiabatic to the isothermal Bulk modulus is equal to 1.

Bulk modulus dimensional analysis:


Dimensional analysis is the process of solving a physical problem by reducing no relevant variables and appealing it to the dimensional homogeneity.
Processing:
Experimental data interpretation
Solve physical problems
Presentation of equations
Establish relative importance
Physical modelling


Bulk modulus,

K=\\frac{-dP}{\\frac{dV}{V}}
P= pressure = [M L-1 T-2]
V=volume= L3
dP=change in pressure= [M L-1 T-2]
dV=change in volume= L3

Application of Bulk modulus:


Diamond- low compressibility-High incompressibility

To find out Compressibility of the material.

Example problems with solutions:


1) A solid ball has initial volume v; it is reduced by 20% when subjected to volumetric stress of 200N/m^2.Find the Bulk modulus of the ball.


Solution:
V1=v, Volumetric strain= Final volume to initial volume *100
Volumetric stress related to volumetric strain=200N/m^2
K= (volumetric stress/Volumetric strain)
= (200/0.02)
=10^4N/m^2

2) The initial pressure of the system is 1.0110^5Pa. The system undergoes a change in pressure to 1.16510^5Pa. Find out the incompressibility of the system.

Solution:
P1=1.0110^5Pa, P2=1.16510^5Pa,
At 20°c change in volume=20%
Bulk modulus=-dP/(dV/V)
=- (1.01×10^5−1.165×10^5)/0.1
=1.55*10^5Pa.

3)5 litres of water is compressed at 20atm.Calculate the volume change in water.


Given:

K of water =20*10^8 N/m^2

Density of mercury=13600 kg/m^3 g=9.81m/s^2

Normal atm.=75cm of mercury

Original volume=5L=510^-3 m^3
Pressure dP=20atm=207510^-2136009.8
Solution:
Volumetric stress= pressure intensity=dp
K = dp/(dv/v)

Change in volume=dpV/K
=5*10^-6 m^3
=5 cc.

Frequently asked questions:


What is the Bulk modulus of granite?
50Gpa.

Can incompressibility be negative:
No.

Bulk modulus formula speed:
The speed of sound depends on the Bulk modulus and density,

v=\\sqrt{\\frac{K}{\\rho }}

Bulk modulus of air at 20 c:
Density of air at 20°C =1.21kg/m^3
Speed of sound=344m/s
So, v=\\sqrt{\\frac{K}{\\rho }}
K can be calculated from the above formula,
344=\\frac{1}{\\sqrt{\\frac{K}{1.21}}}
K=143186.56N/m^2
Hence, K=0.14Mpa

Flexural modulus and incompressibility:
Bulk modulus is the volumetric elasticity and is inversely proportional to the Compressibility. The object having incompressibility deform in all directions when the load is applied from all directions. Flexural modulus is the ability of the material to resist bending. Flexural modulus is the ratio of stress to the strain in flexural deformation.

Modulus of elasticity and incompressibility:

Modulus of elasticity is the ability of the material to resist deformation elastically when applied to external forces. Modulus of elasticity occurs under the elastic deformation region in stress-strain curve. Incompressibility is the volumetric elasticity and is inversely proportional to the Compressibility. The object having volume modulus deform in all directions when the load is applied from all directions

What material has the highest bulk modulus values?
Diamond.

Why is the value of K maximum for a solid but a minimum for gases?
Incompressibility is the resistance to compression of the substance. High pressure is required to compress the solid rather than compressing a gas. Hence the modulus of solid is maximum, and that of gas is low.

If Young’s modulus E is equal to the incompressibility K then what is the value of Poisson’s ratio:

K=E/3(1-2u)
K=E
3(1-2u)=1
1-2u=1/3
u=1/3
So the value of Poisson’s ratio=1/3.

With increase in pressure, Does compressibility decrease or increase ?

As the pressure increases, volume of the body decreases. Decrease in volume gives rise to increase in incompressibility. Incompressibility is the ability to resist the compression of the body. So as it increases the compression of the body decreases. Hence the compressibility of the decreases.

What is the effect of the temperature increase?


As the temperature increases, The resistance to compression decreases.
As the ability of compression of the body decreases, the bulk modulus decreases, leading to an increase in compressibility.

When the incompressibility of a material becomes equal to the shear modulus what would be the Poisson’s ratio:

2G(1+u)=3K(1-2u)
as G=K,
2(1+u)=3(1-2u)
8u=1
u=1/8
Hence the value of Poisson’s ratio=1/8.

What will the velocity of sound in water m/s be if the volume modulus of water is 0.2*10^10 N/m 2:

c=\\sqrt{K\\rho } </em>

c=\\sqrt{\\frac{0.2*10^{10}}{1000}}
c=2*10^6m/s.

To compress a liquid by 10% of its original volume, the pressure required is 2*10^5 N /m^2. What is the K (modulus of the liquid)?

K=\\frac{-dP}{\\frac{dV}{V}}

=-210^5/(-0.9)
=2.22*10^5 N/m^2.

For similar articles, click here.

For more articles, click here.