The average acceleration formula, a = Δv/Δt, is a fundamental concept in physics that describes the rate of change in an object’s velocity over a given time interval. This formula is a crucial tool for understanding and analyzing the motion of objects in one, two, and three dimensions, and it has applications in various fields, including engineering, aerospace, and sports science.
Understanding the Average Acceleration Formula
The average acceleration formula is derived from the basic definition of acceleration, which is the rate of change in velocity. Mathematically, the formula can be expressed as:
a = Δv/Δt
Where:
– a is the average acceleration (in m/s²)
– Δv is the change in velocity (in m/s)
– Δt is the time interval (in s)
The formula tells us that the average acceleration of an object is equal to the change in its velocity divided by the time interval over which that change occurred. This means that if an object’s velocity changes by a certain amount over a specific time period, the average acceleration during that time can be calculated using this formula.
It’s important to note that the average acceleration formula is a vector quantity, meaning it has both magnitude and direction. This means that the acceleration can be positive or negative, depending on whether the object’s velocity is increasing or decreasing.
Calculating Average Acceleration
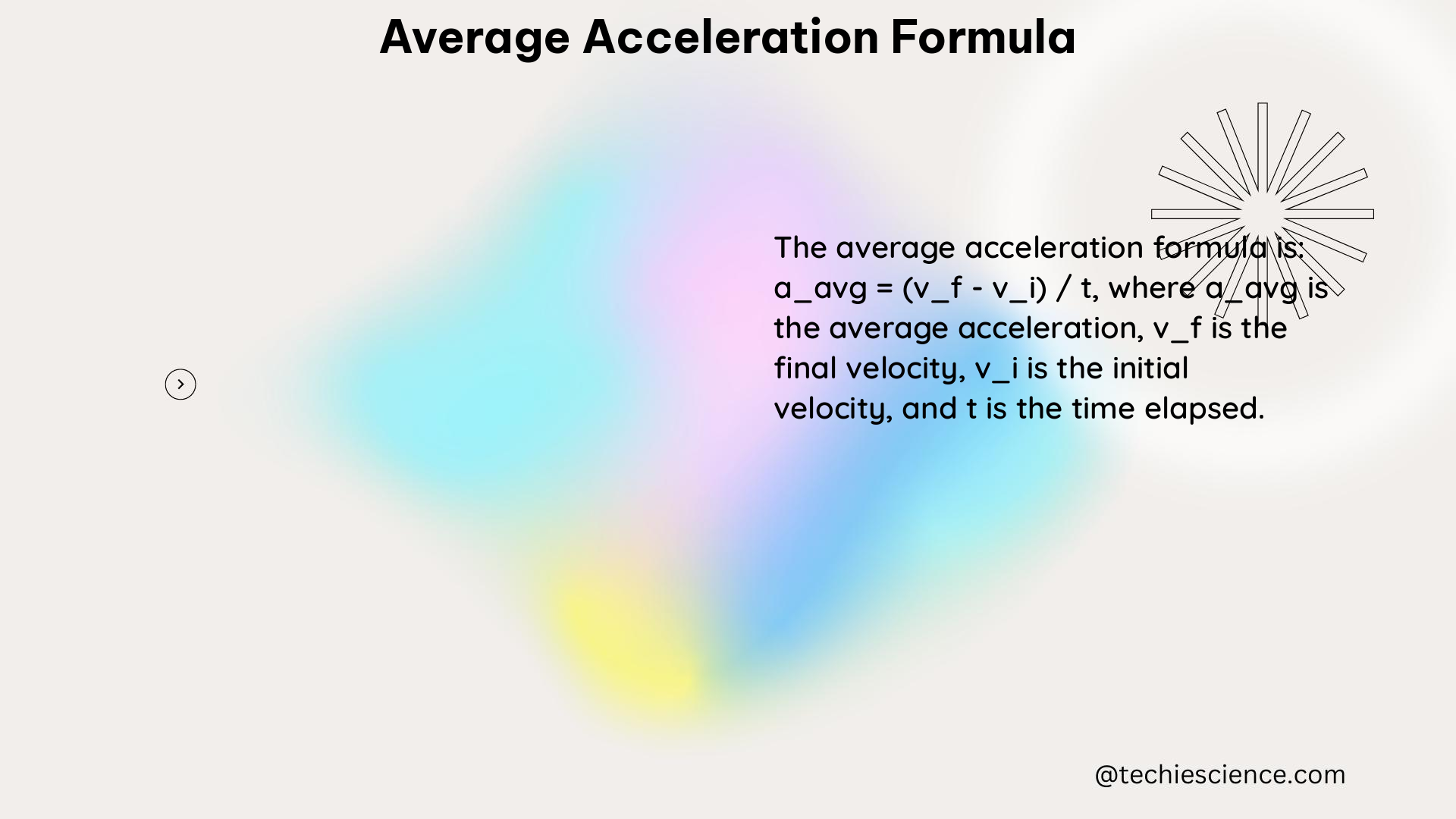
To calculate the average acceleration of an object, you need to know the initial and final velocities, as well as the time interval over which the change in velocity occurred. The formula can then be applied as follows:
a = (v_f - v_i) / Δt
Where:
– a is the average acceleration (in m/s²)
– v_f is the final velocity (in m/s)
– v_i is the initial velocity (in m/s)
– Δt is the time interval (in s)
For example, if a car accelerates from 0 m/s to 60 m/s in 7 seconds, the average acceleration can be calculated as:
a = (60 m/s - 0 m/s) / 7 s = 8.57 m/s²
Similarly, if a bus slows down from 45 m/s to 3 m/s in 1.5 seconds, the average acceleration can be calculated as:
a = (3 m/s - 45 m/s) / 1.5 s = -28 m/s²
Note that the negative value of the acceleration indicates that the bus is decelerating, or slowing down.
Derivation of the Average Acceleration Formula
The average acceleration formula can be derived using integral calculus. The instantaneous acceleration, a(t), is defined as the derivative of the velocity with respect to time:
a(t) = dv/dt
Integrating both sides with respect to time, we get:
∫a(t) dt = ∫dv
Solving this integral, we obtain:
v_f - v_i = ∫a(t) dt
Dividing both sides by the time interval, Δt, we arrive at the average acceleration formula:
a = (v_f - v_i) / Δt
This derivation shows that the average acceleration formula is a direct consequence of the definition of instantaneous acceleration and the fundamental theorem of calculus.
Applications of the Average Acceleration Formula
The average acceleration formula has numerous applications in various fields, including:
-
Kinematics: The average acceleration formula is a key component of kinematics, the study of motion. It is used to analyze the motion of objects in one, two, and three dimensions, and to solve problems involving displacement, velocity, and time.
-
Engineering: In engineering, the average acceleration formula is used to design and analyze the performance of mechanical systems, such as vehicles, machinery, and robotics.
-
Aerospace: In the aerospace industry, the average acceleration formula is used to calculate the acceleration of aircraft and spacecraft during launch, flight, and landing.
-
Sports Science: In sports science, the average acceleration formula is used to analyze the performance of athletes, such as sprinters, jumpers, and cyclists, and to optimize their training and technique.
-
Everyday Life: The average acceleration formula is also relevant in everyday life, such as when analyzing the motion of a car during acceleration or braking, or the motion of a falling object under the influence of gravity.
Numerical Examples and Problems
To further illustrate the application of the average acceleration formula, let’s consider some numerical examples and problems.
Example 1: Acceleration of a Car
A car accelerates from 0 m/s to 80 m/s in 10 seconds. Calculate the average acceleration of the car.
Given:
– Initial velocity, v_i = 0 m/s
– Final velocity, v_f = 80 m/s
– Time interval, Δt = 10 s
Applying the average acceleration formula:
a = (v_f - v_i) / Δt
a = (80 m/s - 0 m/s) / 10 s
a = 8 m/s²
Therefore, the average acceleration of the car is 8 m/s².
Example 2: Deceleration of a Bicycle
A cyclist is traveling at 20 m/s and applies the brakes, slowing down to 5 m/s in 3 seconds. Calculate the average acceleration of the bicycle.
Given:
– Initial velocity, v_i = 20 m/s
– Final velocity, v_f = 5 m/s
– Time interval, Δt = 3 s
Applying the average acceleration formula:
a = (v_f - v_i) / Δt
a = (5 m/s - 20 m/s) / 3 s
a = -5 m/s²
Therefore, the average acceleration of the bicycle is -5 m/s², indicating deceleration.
Problem 1: Falling Object
An object is dropped from a height of 100 m. Assuming the acceleration due to gravity is 9.8 m/s², calculate the average acceleration of the object during the first 2 seconds of its fall.
Given:
– Initial velocity, v_i = 0 m/s
– Time interval, Δt = 2 s
– Acceleration due to gravity, g = 9.8 m/s²
To find the average acceleration, we need to calculate the final velocity using the kinematic equation:
v_f = v_i + at
v_f = 0 + (9.8 m/s²) × (2 s)
v_f = 19.6 m/s
Now, we can apply the average acceleration formula:
a = (v_f - v_i) / Δt
a = (19.6 m/s - 0 m/s) / 2 s
a = 9.8 m/s²
Therefore, the average acceleration of the object during the first 2 seconds of its fall is 9.8 m/s².
Problem 2: Motion of a Projectile
A projectile is launched with an initial velocity of 50 m/s at an angle of 30 degrees above the horizontal. Assuming the acceleration due to gravity is 9.8 m/s², calculate the average acceleration of the projectile during the first 2 seconds of its flight.
Given:
– Initial velocity, v_i = 50 m/s
– Angle of launch, θ = 30 degrees
– Time interval, Δt = 2 s
– Acceleration due to gravity, g = 9.8 m/s²
To find the average acceleration, we need to calculate the final velocity using the kinematic equations for projectile motion:
v_x = v_i cos(θ)
v_y = v_i sin(θ) - gt
v_x = 50 m/s × cos(30°) = 43.3 m/s
v_y = 50 m/s × sin(30°) - (9.8 m/s²) × (2 s) = 25 m/s - 19.6 m/s = 5.4 m/s
v_f = √(v_x^2 + v_y^2)
v_f = √(43.3^2 + 5.4^2)
v_f = 43.8 m/s
Now, we can apply the average acceleration formula:
a = (v_f - v_i) / Δt
a = (43.8 m/s - 50 m/s) / 2 s
a = -3.1 m/s²
Therefore, the average acceleration of the projectile during the first 2 seconds of its flight is -3.1 m/s², indicating a deceleration in the vertical direction due to the influence of gravity.
These examples and problems demonstrate the versatility of the average acceleration formula and its applications in various scenarios. By understanding the formula and its underlying principles, you can effectively analyze and solve problems related to the motion of objects in one, two, and three dimensions.
Conclusion
The average acceleration formula, a = Δv/Δt, is a fundamental concept in physics that describes the rate of change in an object’s velocity over a given time interval. This formula is a crucial tool for understanding and analyzing the motion of objects in one, two, and three dimensions, and it has applications in various fields, including engineering, aerospace, and sports science.
By understanding the derivation of the formula, its applications, and solving numerical examples and problems, you can develop a comprehensive understanding of the average acceleration formula and its role in the study of kinematics and motion. This knowledge will be invaluable in your pursuit of a deeper understanding of physics and its practical applications.
References:
- Kinematics: Displacement, Velocity, and Acceleration
- Derivation of the Average Acceleration Formula
- Applications of the Average Acceleration Formula
- Numerical Problems on Average Acceleration

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.