The Angular Acceleration Formula: A Comprehensive Guide

Summary

The angular acceleration formula, α = (ω₂ – ω₁) / (t₂ – t₁), is a fundamental equation in physics that describes the rate of change of angular velocity, which is the speed of rotation of an object. This formula is essential for understanding and analyzing rotational motion, and it has numerous applications in various fields, including engineering, mechanics, and astronomy.

Understanding the Angular Acceleration Formula

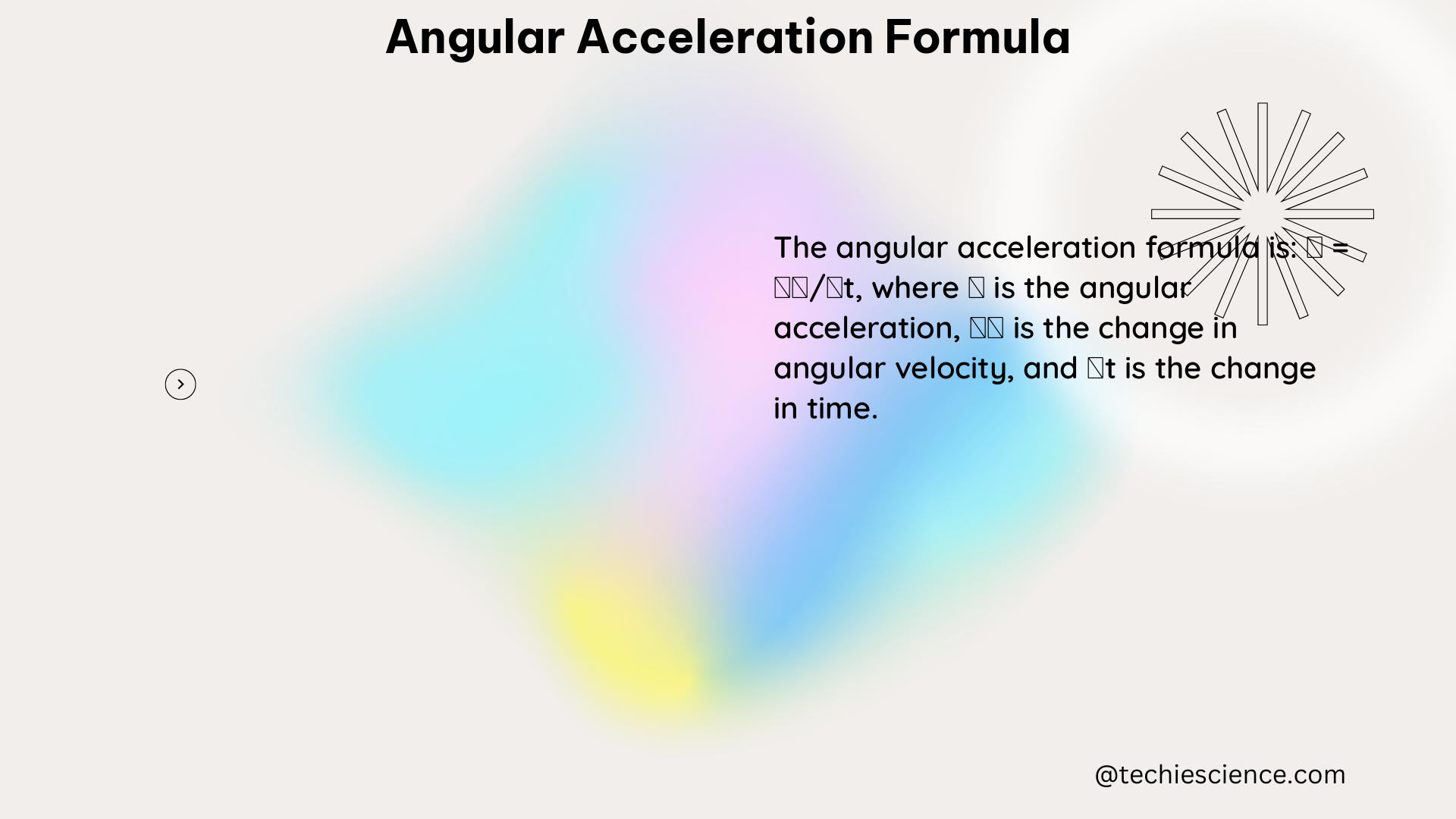
The angular acceleration formula is given by:

α = (ω₂ – ω₁) / (t₂ – t₁)

Where:
– α (alpha) represents the angular acceleration, measured in radians per second squared (rad/s²)
– ω₁ (omega₁) is the initial angular velocity, measured in radians per second (rad/s)
– ω₂ (omega₂) is the final angular velocity, measured in radians per second (rad/s)
– t₁ is the initial time, measured in seconds (s)
– t₂ is the final time, measured in seconds (s)

This formula can be used to calculate the angular acceleration of an object given its initial and final angular velocities, as well as the time interval over which the change in angular velocity occurred.

Derivation of the Angular Acceleration Formula

The angular acceleration formula can be derived from the definition of angular velocity and the concept of the derivative. Angular velocity (ω) is defined as the rate of change of angular position (θ) with respect to time (t):

ω = dθ/dt

Taking the derivative of angular velocity with respect to time, we get:

dω/dt = d²θ/dt²

This expression represents the angular acceleration (α), which is the rate of change of angular velocity with respect to time. Rearranging the terms, we arrive at the angular acceleration formula:

α = dω/dt = d²θ/dt²

Alternatively, the angular acceleration can be expressed in terms of the change in angular velocity and the time interval:

α = (ω₂ – ω₁) / (t₂ – t₁)

This is the angular acceleration formula that we started with.

Relationship between Angular Acceleration and Linear Acceleration

The angular acceleration of an object can also be related to its linear acceleration and the radius of rotation. The relationship is given by:

α = (dv/dt) / r

Where:
– α is the angular acceleration
– dv/dt is the linear acceleration
– r is the radius of rotation

This formula is useful when dealing with rotational motion, as it allows you to connect the linear and angular quantities.

Applications of the Angular Acceleration Formula

The angular acceleration formula has numerous applications in various fields, including:

1. Rotational Dynamics: The formula is used to analyze the motion of objects that rotate around an axis, such as wheels, gears, and flywheels.

2. Mechanics: The formula is essential in the study of torque, which is the rotational equivalent of force, and its relationship with angular acceleration.

3. Engineering: The formula is used in the design and analysis of rotating machinery, such as motors, turbines, and centrifuges.

4. Astronomy: The formula is used to study the rotational motion of celestial bodies, such as planets, moons, and stars.

5. Biomechanics: The formula is used to analyze the rotational motion of body parts, such as the human arm or leg, during various physical activities.

Examples and Numerical Problems

Example 1: Calculating Angular Acceleration of a Car Wheel

A car wheel has a radius of 0.3 meters and a linear acceleration of 5 m/s². Calculate the angular acceleration of the wheel.

Given:
– Radius of the wheel, r = 0.3 m
– Linear acceleration, dv/dt = 5 m/s²

Using the formula:
α = (dv/dt) / r
α = (5 m/s²) / (0.3 m)
α = 16.7 rad/s²

Therefore, the angular acceleration of the car wheel is 16.7 rad/s².

Example 2: Calculating Angular Acceleration of a Fan Blade

A fan blade has a length of 0.2 meters and an angular velocity of 300 rpm. Calculate the angular acceleration of the fan blade.

Given:
– Length of the fan blade, r = 0.2 m
– Initial angular velocity, ω₁ = 300 rpm

First, we need to convert the angular velocity from rpm to rad/s:
ω₁ = (300 rpm) * (2π rad/rev) / (60 s/min) = 31.4 rad/s

Now, we can use the angular acceleration formula:
α = (ω₂ – ω₁) / (t₂ – t₁)

Assuming the fan blade starts from rest (ω₂ = 0 rad/s) and the time interval is 1 second (t₂ – t₁ = 1 s), we get:
α = (0 rad/s – 31.4 rad/s) / (1 s)
α = -31.4 rad/s²

Therefore, the angular acceleration of the fan blade is -31.4 rad/s².

Example 3: Calculating Angular Acceleration of a Merry-Go-Round

A merry-go-round has a radius of 2 meters and an angular velocity of 2 revolutions per minute. Calculate the angular acceleration of the merry-go-round.

Given:
– Radius of the merry-go-round, r = 2 m
– Initial angular velocity, ω₁ = 2 rev/min

First, we need to convert the angular velocity from rev/min to rad/s:
ω₁ = (2 rev/min) * (2π rad/rev) / (60 s/min) = 0.21 rad/s

Assuming the merry-go-round starts from rest (ω₂ = 0 rad/s) and the time interval is 10 seconds (t₂ – t₁ = 10 s), we can use the angular acceleration formula:
α = (ω₂ – ω₁) / (t₂ – t₁)
α = (0 rad/s – 0.21 rad/s) / (10 s)
α = -0.021 rad/s²

Therefore, the angular acceleration of the merry-go-round is -0.021 rad/s².

Conclusion

The angular acceleration formula, α = (ω₂ – ω₁) / (t₂ – t₁), is a fundamental equation in physics that describes the rate of change of angular velocity. This formula is essential for understanding and analyzing rotational motion, and it has numerous applications in various fields, including engineering, mechanics, and astronomy. By understanding the derivation and applications of the angular acceleration formula, you can effectively solve problems and analyze the motion of rotating objects.

References

1. Wikihow. (n.d.). How to Calculate Angular Acceleration. Retrieved from https://www.wikihow.com/Calculate-Angular-Acceleration
2. NCBI. (2021). Angular Acceleration and Its Measurement. Retrieved from https://www.ncbi.nlm.nih.gov/pmc/articles/PMC7830145/
3. Pressbooks. (n.d.). Rotation, Angle, and Angular Velocity. Retrieved from https://pressbooks.bccampus.ca/humanbiomechanics/chapter/6-1-rotation-angle-and-angular-velocity-2/
4. Byjus. (n.d.). Angular Acceleration. Retrieved from https://byjus.com/physics/angular-acceleration/
5. Lumen Learning. (n.d.). Angular Acceleration. Retrieved from https://courses.lumenlearning.com/suny-physics/chapter/10-1-angular-acceleration/