Mastering Amplitude: A Comprehensive Guide for Physics Students

Amplitude is a powerful analytics platform that helps businesses collect, analyze, and activate both quantitative and qualitative data. For physics students, understanding the concept of amplitude and its applications is crucial in various fields, such as wave mechanics, electrical engineering, and signal processing. This comprehensive guide will delve into the technical details of amplitude, providing you with a deep understanding of its principles, formulas, and practical applications.

Understanding Amplitude

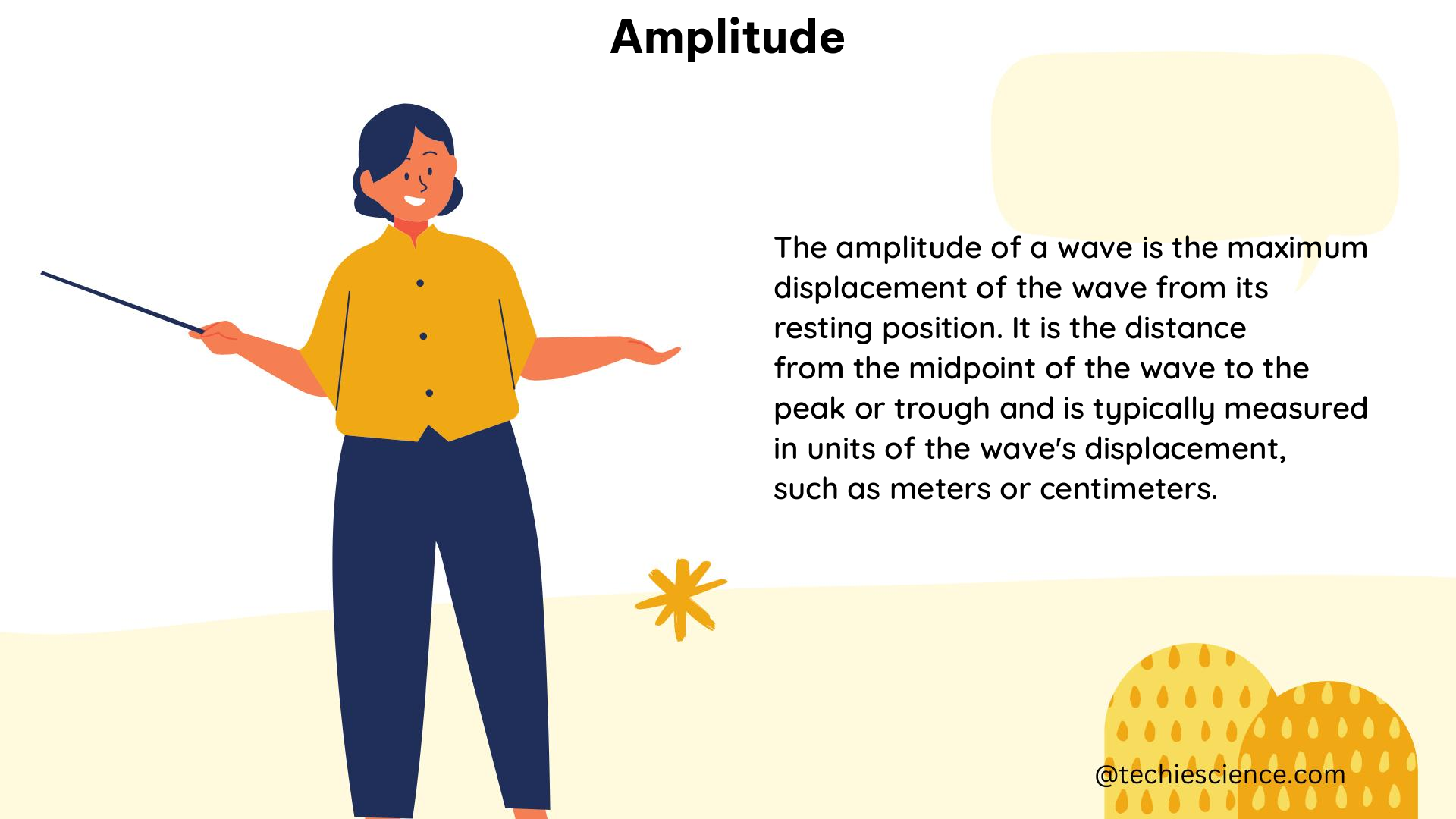
Amplitude, in physics, is a measure of the magnitude or size of a periodic quantity, such as a wave or an oscillation. It represents the maximum displacement or deviation from the average or resting position of a vibrating or oscillating system. Amplitude is a fundamental concept in various areas of physics, including:

1. Wave Mechanics: In wave mechanics, amplitude represents the maximum displacement of a wave from its equilibrium position. It is a crucial parameter in the study of wave propagation, interference, and diffraction.

2. Electrical Engineering: In electrical circuits, amplitude refers to the maximum value of an alternating current (AC) or voltage waveform. It is essential in the analysis and design of electronic circuits and systems.

3. Signal Processing: In signal processing, amplitude is a measure of the strength or magnitude of a signal. It is used in the analysis and manipulation of various types of signals, such as audio, video, and communication signals.

Quantifying Amplitude

Amplitude can be quantified using various mathematical expressions and formulas. Here are some of the key formulas and their applications:

1. Sine Wave Amplitude: The amplitude of a sine wave is the maximum displacement of the wave from its equilibrium position. The formula for the amplitude of a sine wave is:

A = A_max

where A is the amplitude and A_max is the maximum displacement.

1. Amplitude of a Harmonic Oscillator: The amplitude of a harmonic oscillator, such as a mass-spring system or a pendulum, is the maximum displacement from the equilibrium position. The formula for the amplitude of a harmonic oscillator is:

A = x_max

where A is the amplitude and x_max is the maximum displacement.

1. Amplitude of an Electromagnetic Wave: The amplitude of an electromagnetic wave, such as a radio wave or a light wave, is the maximum value of the electric or magnetic field. The formula for the amplitude of an electromagnetic wave is:

A = E_max or A = B_max

where A is the amplitude, E_max is the maximum electric field, and B_max is the maximum magnetic field.

1. Amplitude of a Voltage or Current Waveform: The amplitude of a voltage or current waveform in an electrical circuit is the maximum value of the waveform. The formula for the amplitude of a voltage or current waveform is:

A = V_max or A = I_max

where A is the amplitude, V_max is the maximum voltage, and I_max is the maximum current.

Amplitude Measurement Techniques

Measuring amplitude accurately is crucial in various applications. Here are some common techniques used to measure amplitude:

1. Oscilloscope: An oscilloscope is a widely used instrument for measuring the amplitude of electrical signals. It displays the waveform of the signal and allows the user to measure the amplitude directly from the screen.

2. Voltmeter: A voltmeter is a device used to measure the amplitude of a voltage waveform. It can be used to measure the peak-to-peak or root-mean-square (RMS) value of the waveform.

3. Ammeter: An ammeter is a device used to measure the amplitude of a current waveform. It can be used to measure the peak-to-peak or RMS value of the current.

4. Laser Interferometry: Laser interferometry is a technique used to measure the amplitude of small displacements, such as those in micro-electromechanical systems (MEMS) or atomic force microscopes (AFM).

5. Accelerometer: An accelerometer is a device used to measure the amplitude of vibrations or accelerations. It can be used to measure the amplitude of mechanical oscillations or seismic waves.

Amplitude Applications in Physics

Amplitude has a wide range of applications in various fields of physics. Here are some examples:

1. Wave Mechanics: Amplitude is a crucial parameter in the study of wave propagation, interference, and diffraction. It is used to analyze the behavior of waves, such as sound waves, electromagnetic waves, and water waves.

2. Electrical Engineering: Amplitude is essential in the analysis and design of electrical circuits and systems. It is used to characterize the behavior of alternating current (AC) signals, such as those in power transmission, communication systems, and electronic devices.

3. Signal Processing: Amplitude is a fundamental parameter in signal processing, where it is used to analyze and manipulate various types of signals, such as audio, video, and communication signals.

4. Quantum Mechanics: In quantum mechanics, the amplitude of a wavefunction represents the probability of finding a particle in a particular state. It is a crucial concept in the study of quantum phenomena, such as quantum tunneling and quantum entanglement.

5. Astrophysics: Amplitude is used in the study of astronomical phenomena, such as the brightness variations of stars, the oscillations of the Sun, and the detection of gravitational waves.

Amplitude Numerical Problems and Examples

To further solidify your understanding of amplitude, let’s explore some numerical problems and examples:

1. Sine Wave Amplitude: A sine wave has a maximum displacement of 5 volts. Calculate the amplitude of the sine wave.

“`
Given:
Maximum displacement (A_max) = 5 volts

Amplitude (A) = A_max = 5 volts
“`

1. Amplitude of a Harmonic Oscillator: A mass-spring system has a maximum displacement of 2 centimeters from its equilibrium position. Calculate the amplitude of the harmonic oscillator.

“`
Given:
Maximum displacement (x_max) = 2 cm

Amplitude (A) = x_max = 2 cm
“`

1. Amplitude of an Electromagnetic Wave: An electromagnetic wave has a maximum electric field of 10 volts per meter. Calculate the amplitude of the electromagnetic wave.

“`
Given:
Maximum electric field (E_max) = 10 V/m

Amplitude (A) = E_max = 10 V/m
“`

1. Amplitude of a Voltage Waveform: A voltage waveform in an electrical circuit has a peak-to-peak value of 20 volts. Calculate the amplitude of the voltage waveform.

“`
Given:
Peak-to-peak value = 20 volts

Amplitude (A) = V_max = 10 volts (since the peak-to-peak value is twice the amplitude)
“`

1. Amplitude Measurement using an Oscilloscope: An oscilloscope is used to measure the amplitude of a voltage waveform. The waveform is displayed on the oscilloscope screen, and the vertical scale is set to 2 volts per division. The waveform spans 4 divisions in the vertical direction. Calculate the amplitude of the voltage waveform.

“`
Given:
Vertical scale: 2 volts per division
Vertical span: 4 divisions

Amplitude (A) = Vertical span × Vertical scale
Amplitude (A) = 4 divisions × 2 volts/division = 8 volts
“`

These examples demonstrate the application of amplitude formulas and measurement techniques in various physics scenarios. By working through these problems, you can develop a deeper understanding of the concept of amplitude and its practical implications.

Conclusion

Amplitude is a fundamental concept in physics, with applications in wave mechanics, electrical engineering, and signal processing. This comprehensive guide has provided you with a detailed understanding of amplitude, including its mathematical formulas, measurement techniques, and practical applications. By mastering the concepts and problem-solving skills presented in this guide, you will be well-equipped to tackle amplitude-related challenges in your physics studies and research.

References

1. https://www.physicsclassroom.com/class/waves/Lesson-1/Amplitude
2. https://www.electronics-tutorials.ws/accircuits/ac-waveforms.html
3. https://www.sciencedirect.com/topics/engineering/amplitude-measurement
4. https://www.electronics-tutorials.ws/accircuits/rms-voltage.html
5. https://www.electronics-tutorials.ws/accircuits/peak-to-peak-voltage.html