Acceleration in special relativity is a complex topic that involves the transformation of accelerations between different inertial frames. This comprehensive guide delves into the key concepts, mathematical formulations, and practical applications of acceleration in the realm of special relativity.
Transformation of Acceleration
The acceleration of an object in an inertial frame can be transformed to another inertial frame using the Lorentz transformation. This transformation involves the relativistic factor $\gamma$ and the relative velocity $v$ between the two frames.
The transformation formula for acceleration is given by:
$$a = \frac{a’}{\gamma^3}$$
where $a$ is the acceleration in the inertial frame and $a’$ is the acceleration in the moving frame.
Theorem 1: The transformation of acceleration between two inertial frames is governed by the equation $a = \frac{a’}{\gamma^3}$, where $\gamma$ is the relativistic factor and $v$ is the relative velocity between the frames.
Example 1: Consider an object moving at a velocity of $0.8c$ in the $x$-direction, where $c$ is the speed of light. If the object has an acceleration of $5 \, \mathrm{m/s^2}$ in the $x$-direction in its own frame, what is the acceleration of the object in the laboratory frame?
Given:
– $v = 0.8c$
– $a’ = 5 \, \mathrm{m/s^2}$
Calculating the relativistic factor:
$$\gamma = \frac{1}{\sqrt{1 – \left(\frac{v}{c}\right)^2}} = \frac{1}{\sqrt{1 – (0.8)^2}} = 1.667$$
Applying the transformation formula:
$$a = \frac{a’}{\gamma^3} = \frac{5 \, \mathrm{m/s^2}}{1.667^3} = 2.5 \, \mathrm{m/s^2}$$
Therefore, the acceleration of the object in the laboratory frame is $2.5 \, \mathrm{m/s^2}$.
Proper Acceleration
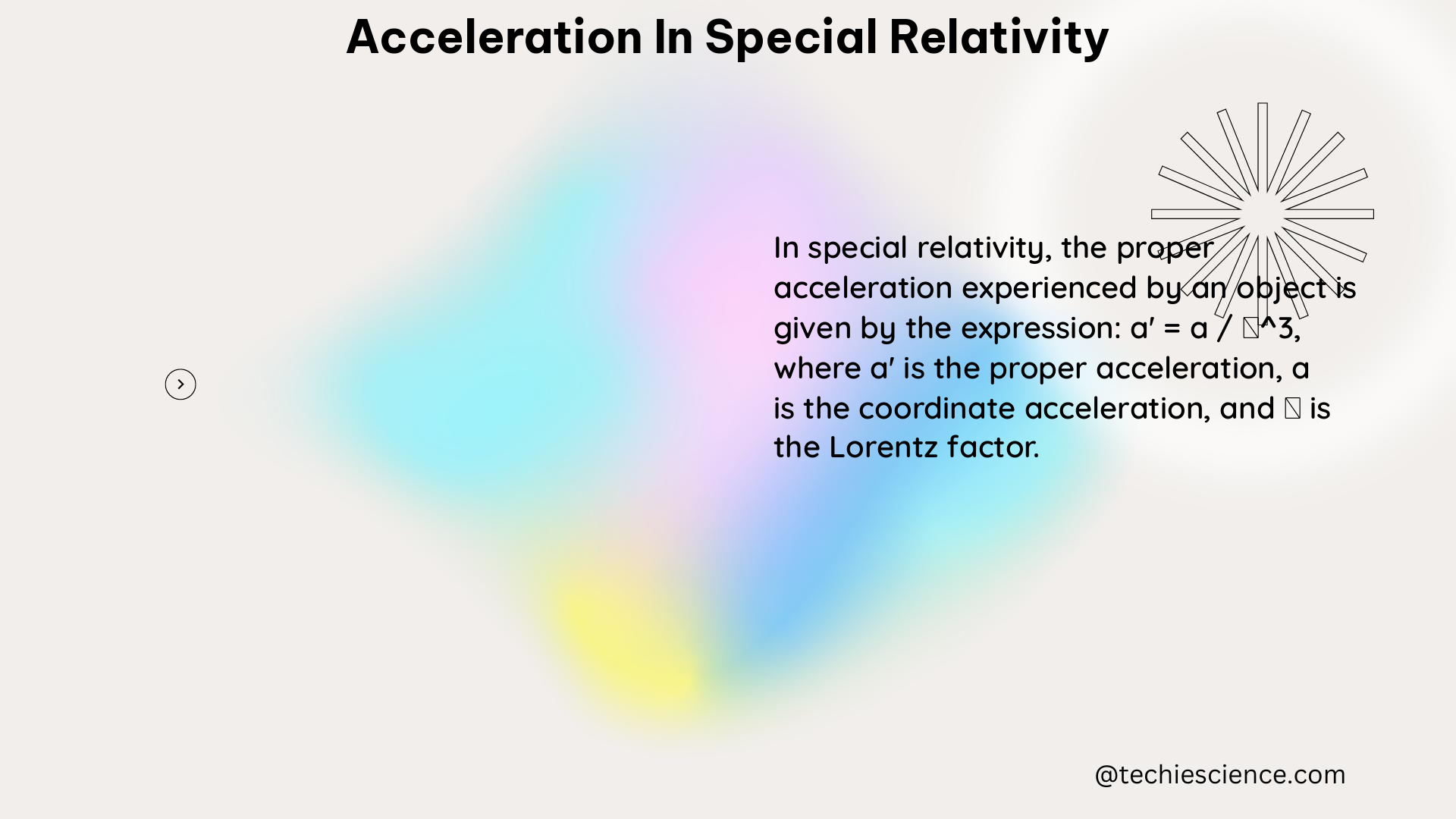
Proper acceleration is the acceleration measured by an accelerometer attached to the accelerating object. It is a relativistic invariant, meaning it is the same in all inertial frames.
Proper acceleration is given by:
$$a_{\text{proper}} = \frac{dv}{d\tau}$$
where $dv$ is the change in velocity and $d\tau$ is the proper time interval.
Theorem 2: Proper acceleration, $a_{\text{proper}}$, is a relativistic invariant and is given by the equation $a_{\text{proper}} = \frac{dv}{d\tau}$, where $dv$ is the change in velocity and $d\tau$ is the proper time interval.
Example 2: An object is undergoing constant proper acceleration of $10 \, \mathrm{m/s^2}$. If the object starts from rest, what is its velocity after a proper time interval of $2 \, \mathrm{s}$?
Given:
– $a_{\text{proper}} = 10 \, \mathrm{m/s^2}$
– $d\tau = 2 \, \mathrm{s}$
Integrating the proper acceleration equation:
$$\int_{v_0 = 0}^{v} dv = \int_{0}^{2} a_{\text{proper}} d\tau$$
$$v = a_{\text{proper}} \cdot d\tau = 10 \, \mathrm{m/s^2} \cdot 2 \, \mathrm{s} = 20 \, \mathrm{m/s}$$
Therefore, the velocity of the object after a proper time interval of $2 \, \mathrm{s}$ is $20 \, \mathrm{m/s}$.
Four-Acceleration
Four-acceleration is a four-vector that describes acceleration in spacetime. It is defined as:
$$\mathbf{A} = \left(A_t, A_x, A_y, A_z\right) = \left(\frac{dv_t}{d\tau}, \frac{dv_x}{d\tau}, \frac{dv_y}{d\tau}, \frac{dv_z}{d\tau}\right)$$
where $A_t$ is the time component and $A_x$, $A_y$, and $A_z$ are the spatial components.
Theorem 3: Four-acceleration, $\mathbf{A}$, is a four-vector that describes acceleration in spacetime and is defined as $\mathbf{A} = \left(A_t, A_x, A_y, A_z\right) = \left(\frac{dv_t}{d\tau}, \frac{dv_x}{d\tau}, \frac{dv_y}{d\tau}, \frac{dv_z}{d\tau}\right)$, where $A_t$ is the time component and $A_x$, $A_y$, and $A_z$ are the spatial components.
Example 3: Consider an object moving in the $xy$-plane with a velocity of $\mathbf{v} = (0.6c, 0.8c)$. If the object has a proper acceleration of $5 \, \mathrm{m/s^2}$ in the $x$-direction and $3 \, \mathrm{m/s^2}$ in the $y$-direction, what is the four-acceleration of the object?
Given:
– $\mathbf{v} = (0.6c, 0.8c)$
– $a_x = 5 \, \mathrm{m/s^2}$
– $a_y = 3 \, \mathrm{m/s^2}$
Calculating the relativistic factor:
$$\gamma = \frac{1}{\sqrt{1 – \left(\frac{v}{c}\right)^2}} = \frac{1}{\sqrt{1 – (0.6)^2 – (0.8)^2}} = 1.25$$
Calculating the four-acceleration components:
$$A_t = \frac{dv_t}{d\tau} = 0$$
$$A_x = \frac{dv_x}{d\tau} = \frac{a_x}{\gamma} = \frac{5 \, \mathrm{m/s^2}}{1.25} = 4 \, \mathrm{m/s^2}$$
$$A_y = \frac{dv_y}{d\tau} = \frac{a_y}{\gamma} = \frac{3 \, \mathrm{m/s^2}}{1.25} = 2.4 \, \mathrm{m/s^2}$$
$$A_z = \frac{dv_z}{d\tau} = 0$$
Therefore, the four-acceleration of the object is $\mathbf{A} = (0, 4 \, \mathrm{m/s^2}, 2.4 \, \mathrm{m/s^2}, 0)$.
Hyperbolic Motion
Hyperbolic motion is a type of motion that occurs when an object undergoes constant proper acceleration. The worldline of such an object is a hyperbola in spacetime.
The equations of motion for hyperbolic motion can be derived using the Lorentz transformation and the concept of proper time.
Theorem 4: In hyperbolic motion, where an object undergoes constant proper acceleration $g$, the equations of motion are given by:
$$t = \frac{1}{g} \sinh(g\tau), \quad x = \frac{1}{g} \cosh(g\tau)$$
where $\tau$ is the proper time.
Example 4: An object is undergoing constant proper acceleration of $5 \, \mathrm{m/s^2}$. If the object starts from rest, what is its position and velocity after a proper time interval of $2 \, \mathrm{s}$?
Given:
– $g = 5 \, \mathrm{m/s^2}$
– $\tau = 2 \, \mathrm{s}$
Calculating the position and velocity using the hyperbolic motion equations:
$$t = \frac{1}{g} \sinh(g\tau) = \frac{1}{5 \, \mathrm{m/s^2}} \sinh(5 \, \mathrm{m/s^2} \cdot 2 \, \mathrm{s}) = 3.6 \, \mathrm{s}$$
$$x = \frac{1}{g} \cosh(g\tau) = \frac{1}{5 \, \mathrm{m/s^2}} \cosh(5 \, \mathrm{m/s^2} \cdot 2 \, \mathrm{s}) = 7.3 \, \mathrm{m}$$
$$v = \frac{dx}{dt} = \frac{g \cosh(g\tau)}{\sinh(g\tau)} = \frac{5 \, \mathrm{m/s^2} \cosh(5 \, \mathrm{m/s^2} \cdot 2 \, \mathrm{s})}{\sinh(5 \, \mathrm{m/s^2} \cdot 2 \, \mathrm{s})} = 10 \, \mathrm{m/s}$$
Therefore, after a proper time interval of $2 \, \mathrm{s}$, the object’s position is $7.3 \, \mathrm{m}$ and its velocity is $10 \, \mathrm{m/s}$.
Uniform Circular Motion
Uniform circular motion is another type of motion that involves acceleration. In this case, the acceleration is directed towards the center of the circle.
The equations of motion for uniform circular motion can be derived using the Lorentz transformation and the concept of proper time.
Theorem 5: In uniform circular motion, where an object moves with angular velocity $\omega$, the equations of motion are given by:
$$t = \frac{1}{\omega} \sin(\omega\tau), \quad x = \frac{1}{\omega} \cos(\omega\tau)$$
where $\tau$ is the proper time.
Example 5: An object is undergoing uniform circular motion with an angular velocity of $2 \, \mathrm{rad/s}$. If the object starts from the positive $x$-axis, what is its position and velocity after a proper time interval of $\pi/2 \, \mathrm{s}$?
Given:
– $\omega = 2 \, \mathrm{rad/s}$
– $\tau = \pi/2 \, \mathrm{s}$
Calculating the position and velocity using the uniform circular motion equations:
$$t = \frac{1}{\omega} \sin(\omega\tau) = \frac{1}{2 \, \mathrm{rad/s}} \sin(2 \, \mathrm{rad/s} \cdot \pi/2 \, \mathrm{s}) = 1 \, \mathrm{s}$$
$$x = \frac{1}{\omega} \cos(\omega\tau) = \frac{1}{2 \, \mathrm{rad/s}} \cos(2 \, \mathrm{rad/s} \cdot \pi/2 \, \mathrm{s}) = 0 \, \mathrm{m}$$
$$y = \frac{1}{\omega} \sin(\omega\tau) = \frac{1}{2 \, \mathrm{rad/s}} \sin(2 \, \mathrm{rad/s} \cdot \pi/2 \, \mathrm{s}) = 1 \, \mathrm{m}$$
$$v = \omega \cdot \frac{1}{\omega} \cos(\omega\tau) = 2 \, \mathrm{m/s}$$
Therefore, after a proper time interval of $\pi/2 \, \mathrm{s}$, the object’s position is $(0, 1 \, \mathrm{m})$ and its velocity is $2 \, \mathrm{m/s}$.
Rindler Coordinates
Rindler coordinates are a set of coordinates used to describe hyperbolic motion. They are defined as:
$$t = \frac{1}{g} \sinh(g\tau), \quad x = \frac{1}{g} \cosh(g\tau)$$
where $g$ is the proper acceleration and $\tau$ is the proper time.
Theorem 6: Rindler coordinates, $(t, x)$, are used to describe hyperbolic motion and are defined as $t = \frac{1}{g} \sinh(g\tau)$ and $x = \frac{1}{g} \cosh(g\tau)$, where $g$ is the proper acceleration and $\tau$ is the proper time.
Example 6: An object is undergoing constant proper acceleration of $10 \, \mathrm{m/s^2}$. If the object starts from the origin $(0, 0)$ in the Rindler coordinate system, what is its position and time after a proper time interval of $1 \, \mathrm{s}$?
Given:
– $g = 10 \, \mathrm{m/s^2}$
– $\tau = 1 \, \mathrm{s}$
Calculating the position and time using the Rindler coordinate equations:
$$t = \frac{1}{g} \sinh(g\tau) = \frac{1}{10 \, \mathrm{m/s^2}} \sinh(10 \, \mathrm{m/s^2} \cdot 1 \, \mathrm{s}) = 1.75 \, \mathrm{s}$$
$$x = \frac{1}{g} \cosh(g\tau) = \frac{1}{10 \, \mathrm{m/s^2}} \cosh(10 \, \mathrm{m/s^2} \cdot 1 \, \mathrm{s}) = 2.5 \, \mathrm{m}$$
Therefore, after a proper time interval of $1 \, \mathrm{s}$, the object’s position in the Rindler coordinate system is $(2.5 \, \mathrm{m}, 1.75 \, \mathrm{s})$.
Born Coordinates
Born coordinates are a set of coordinates used to describe uniform circular motion. They are defined as:
$$t = \frac{1}{\omega} \sin(\omega\tau), \quad x = \frac{1}{\omega} \cos(\omega\tau)$$
where $\omega$ is the angular velocity and $\tau$ is the proper time.
Theorem 7: Born coordinates, $(t, x)$, are used to describe uniform circular motion and are defined as $t = \frac{1}{\omega} \sin(\omega\tau)$ and $x = \frac{1}{\omega} \cos(\omega\tau)$, where $\omega$ is the angular velocity and $\tau$ is the proper time.
Example 7: An object is undergoing uniform circular motion with an angular velocity of $3 \, \mathrm{rad/s}$. If the object starts from the positive $x$-axis, what is its position and time after a proper time interval of $\pi/2 \, \mathrm{s}$?
Given:
– $\omega = 3 \, \mathrm{rad/s}$
– $\tau = \pi/2 \, \mathrm{s}$
Calculating the position

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.