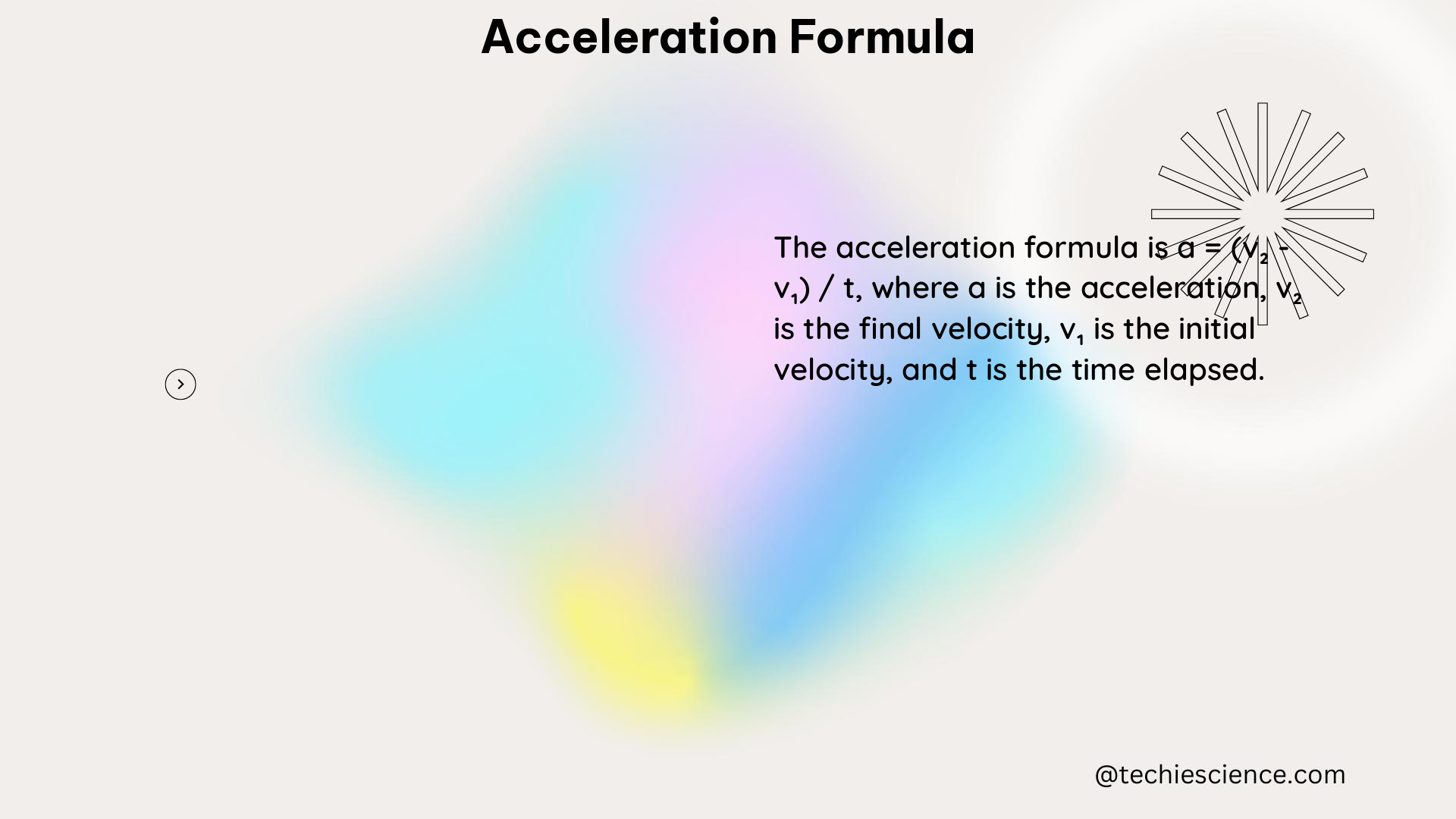
The acceleration formula is a fundamental concept in physics that describes the rate of change in an object’s velocity over time. It is given by the equation a = (vf - vi)/t, where a is acceleration, vf is final velocity, vi is initial velocity, and t is time. The SI unit of acceleration is meters per second squared (m/s^2).
Understanding the Acceleration Formula
The acceleration formula is derived from the basic principles of motion and the relationship between velocity, time, and distance. The formula can be used to calculate the acceleration of an object given its initial and final velocities, as well as the time interval over which the change in velocity occurred.
The formula can be rearranged to solve for different variables:
- Acceleration:
a = (vf - vi)/t - Final Velocity:
vf = vi + at - Initial Velocity:
vi = vf - at - Time:
t = (vf - vi)/a
These rearrangements allow the formula to be applied to a variety of physics problems, from analyzing the motion of a car to understanding the acceleration of a falling object.
Calculating Acceleration: Examples and Numerical Problems
Let’s explore some examples and numerical problems to better understand the application of the acceleration formula.
Example 1: Calculating the Average Acceleration of a Car
A car accelerates from 0 m/s to 60 m/s over a time interval of 7 seconds. Calculate the average acceleration of the car.
Given:
– Initial velocity (vi) = 0 m/s
– Final velocity (vf) = 60 m/s
– Time (t) = 7 s
Using the acceleration formula:
a = (vf - vi)/t
a = (60 m/s - 0 m/s)/7 s
a = 60 m/s / 7 s
a = 8.57 m/s^2
Therefore, the average acceleration of the car is 8.57 m/s^2.
Example 2: Calculating the Average Acceleration of a Bus
A bus decelerates from 45 m/s to 3 m/s over a time interval of 1.5 seconds. Calculate the average acceleration of the bus.
Given:
– Initial velocity (vi) = 45 m/s
– Final velocity (vf) = 3 m/s
– Time (t) = 1.5 s
Using the acceleration formula:
a = (vf - vi)/t
a = (3 m/s - 45 m/s)/1.5 s
a = -28 m/s^2
The negative sign indicates that the bus is decelerating.
Therefore, the average acceleration of the bus is -28 m/s^2.
Numerical Problem 1: Calculating Acceleration from Accelerometer Data
An accelerometer measures the acceleration of an object in three axes (x, y, and z). The accelerometer data for a high-velocity, low-amplitude motion of a hand is as follows:
- Acceleration in x-axis: 2.5 m/s^2
- Acceleration in y-axis: -1.8 m/s^2
- Acceleration in z-axis: 0.9 m/s^2
Calculate the total acceleration of the hand.
To calculate the total acceleration, we use the formula:
a_total = sqrt(a_x^2 + a_y^2 + a_z^2)
Substituting the values:
a_total = sqrt((2.5 m/s^2)^2 + (-1.8 m/s^2)^2 + (0.9 m/s^2)^2)
a_total = sqrt(6.25 + 3.24 + 0.81)
a_total = sqrt(10.3)
a_total = 3.21 m/s^2
Therefore, the total acceleration of the hand is 3.21 m/s^2.
Related Concepts and Graphs
In addition to the acceleration formula, there are other related concepts and graphs that are important to understand in the study of physics.
Average Acceleration
Average acceleration is the rate of change of velocity over a fixed period of time. It is calculated using the formula:
a_avg = (vf - vi)/t
This formula is the same as the acceleration formula, but it represents the average acceleration over the entire time interval, rather than the instantaneous acceleration at a specific point in time.
Angular Acceleration
Angular acceleration is the rate of change of angular velocity over time. It is calculated using the formula:
α = (ω_f - ω_i)/t
where α is angular acceleration, ω_f is the final angular velocity, ω_i is the initial angular velocity, and t is the time interval.
Acceleration-Time Graph
An acceleration-time graph is a graphical representation of the variation of acceleration with time. The graph can be used to analyze the motion of an object and understand its acceleration characteristics. The slope of the acceleration-time graph represents the rate of change of acceleration, which is known as jerk.
Conclusion
The acceleration formula is a fundamental concept in physics that describes the rate of change in an object’s velocity over time. By understanding the formula and its applications, as well as related concepts and graphs, physics students can develop a deeper understanding of the principles of motion and apply them to a wide range of problems and scenarios.
References
- Average Acceleration Formula – GeeksforGeeks, https://www.geeksforgeeks.org/average-acceleration-formula/
- quant lit 2 acceleration formula – YouTube, https://www.youtube.com/watch?v=NfHIQGkcEAE
- Determining the Acceleration of an Object – Lesson – Study.com, https://study.com/academy/lesson/determining-the-acceleration-of-an-object.html
- Acceleration load, acceleration density and acceleration density index, https://support.catapultsports.com/hc/en-us/articles/360001559976-Acceleration-load-acceleration-density-and-acceleration-density-index
- Using accelerometer data to calculate distance – Physics Forums, https://www.physicsforums.com/threads/using-accelerometer-data-to-calculate-distance.845174/

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.