Mastering Kinetic Energy: A Comprehensive Guide to Finding It Using Distance and Force

Summary

Kinetic energy is a fundamental concept in physics, and understanding how to calculate it using distance and force is crucial for many applications. This comprehensive guide will take you through the step-by-step process of finding kinetic energy, starting from the work-energy theorem and the formulas for work and kinetic energy. You’ll learn how to rearrange these equations to solve for velocity, change in kinetic energy, and distance traveled. Additionally, we’ll dive into a detailed example to solidify your understanding of the concepts. By the end of this article, you’ll be equipped with the knowledge and skills to confidently tackle kinetic energy problems involving distance and force.

The Work-Energy Theorem

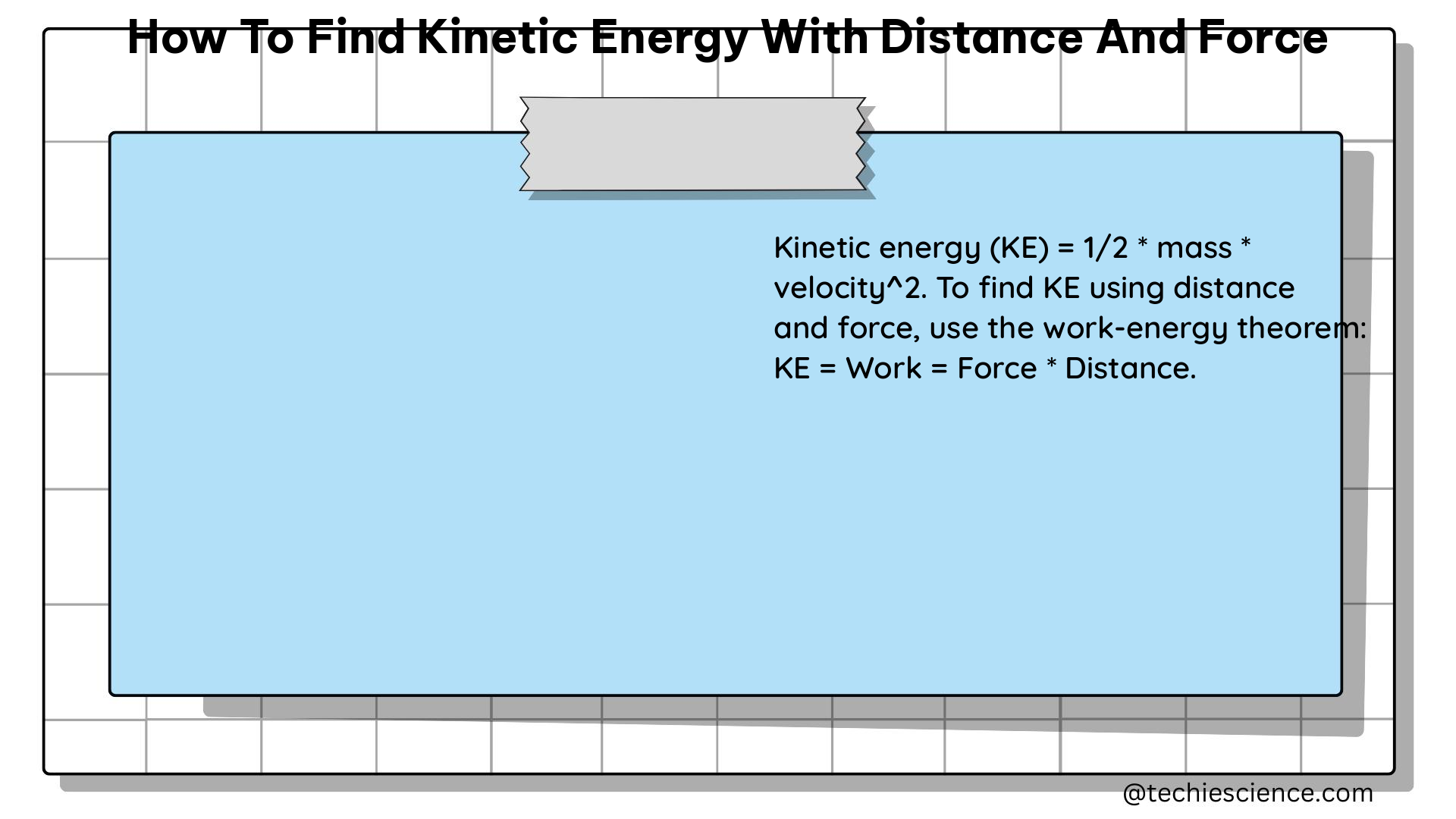
The work-energy theorem is the foundation for finding kinetic energy using distance and force. It states that the work done on an object is equal to the change in its kinetic energy. The formula for work is given by:

Work (W) = Force (F) × Distance (d) × cos(θ)

where θ is the angle between the force and the direction of motion. If the force and distance are in the same direction, then cos(θ) is equal to 1.

The Formula for Kinetic Energy

The formula for kinetic energy is given by:

Kinetic Energy (KE) = 1/2 × Mass (m) × Velocity (v)^2

We can rearrange this formula to solve for velocity:

v = sqrt(2 × KE / m)

Finding Kinetic Energy at a Certain Point

To find the kinetic energy at a certain point, we can use the work-energy theorem and the formula for kinetic energy. First, we need to find the work done on the object. We can do this by multiplying the force by the distance. Then, we can use the work-energy theorem to find the change in kinetic energy:

ΔKE = W

If we know the initial kinetic energy (KEi) and the final kinetic energy (KEf), we can find the change in kinetic energy by subtracting the initial kinetic energy from the final kinetic energy:

ΔKE = KEf – KEi

Once we have the change in kinetic energy, we can use the formula for kinetic energy to find the final velocity:

v = sqrt(2 × (KEi + ΔKE) / m)

Finding the Distance Traveled

If we want to find the distance traveled, we can use the work-energy theorem again. We know that the work done on the object is equal to the change in kinetic energy, so we can set the work equation equal to the change in kinetic energy equation and solve for the distance:

F × d × cos(θ) = 1/2 × Mass (m) × (vf^2 – vi^2)

where vi is the initial velocity and vf is the final velocity.

Example Problem

Let’s consider a car with a mass of 1500 kg. The car is moving at a speed of 20 m/s when a braking force of 5000 N is applied. We want to find the kinetic energy of the car when it has traveled 20 meters.

1. Find the work done on the car:
   W = F × d × cos(θ)
   W = 5000 N × 20 m × 1 (since the force and distance are in the same direction)
   W = 100,000 J

2. Find the change in kinetic energy:
   ΔKE = W
   ΔKE = 100,000 J

3. Find the initial kinetic energy (KEi):
   KEi = 1/2 × Mass (m) × Vi^2
   KEi = 1/2 × 1500 kg × (20 m/s)^2
   KEi = 300,000 J

4. Find the final kinetic energy (KEf):
   KEf = KEi + ΔKE
   KEf = 300,000 J + 100,000 J
   KEf = 400,000 J

5. Find the final velocity (vf):
   vf = sqrt(2 × KEf / m)
   vf = sqrt(2 × 400,000 J / 1500 kg)
   vf = 28.28 m/s

6. Find the distance traveled (d):
   F × d × cos(θ) = 1/2 × Mass (m) × (vf^2 – vi^2)
   5000 N × d × 1 = 1/2 × 1500 kg × ((28.28 m/s)^2 – (20 m/s)^2)
   d = 20 m

Therefore, the kinetic energy of the car when it has traveled 20 meters is 400,000 J.

Conclusion

In this comprehensive guide, we have explored the step-by-step process of finding kinetic energy using distance and force. We started with the work-energy theorem and the formulas for work and kinetic energy, and then delved into the rearrangement of these equations to solve for velocity, change in kinetic energy, and distance traveled. The detailed example problem provided a practical application of the concepts, solidifying your understanding of how to tackle kinetic energy problems involving distance and force.

Remember, the key to mastering this topic is to practice applying the formulas and principles to a variety of scenarios. Continuously challenging yourself with new problems will help you develop a deeper understanding and the ability to confidently solve kinetic energy problems in the future.

References:

1. Impact Forces: Calculations & Formulas – StudySmarter. (n.d.). Retrieved from https://www.studysmarter.co.uk/explanations/physics/force/impact-forces/
2. Practice Applying Velocity & Energy Formulas. (n.d.). Retrieved from https://study.com/academy/lesson/practice-applying-velocity-energy-formulas.html
3. Analysis of Situations Involving External Forces. (n.d.). Retrieved from https://www.physicsclassroom.com/class/energy/Lesson-2/Analysis-of-Situations-Involving-External-Forces
4. How to Use the Work-Energy Theorem to Calculate the Distance Traveled by an Object: Explanation & Example. (n.d.). Retrieved from https://study.com/skill/learn/how-to-use-the-work-energy-theorem-to-calculate-the-distance-traveled-by-an-object-explanation.html